【C++历练之路】二叉搜索树的学习应用及其实现

W...Y的主页 😊
代码仓库分享💕

前言🍔:
我们之前学过一些查找关键数据的办法,排序+二分查找。但是这种方法的插入的时间复杂的太高,今天我们来学习一个更好的办法来应对数据查找——二叉搜索树。
目录
1. 二叉搜索树
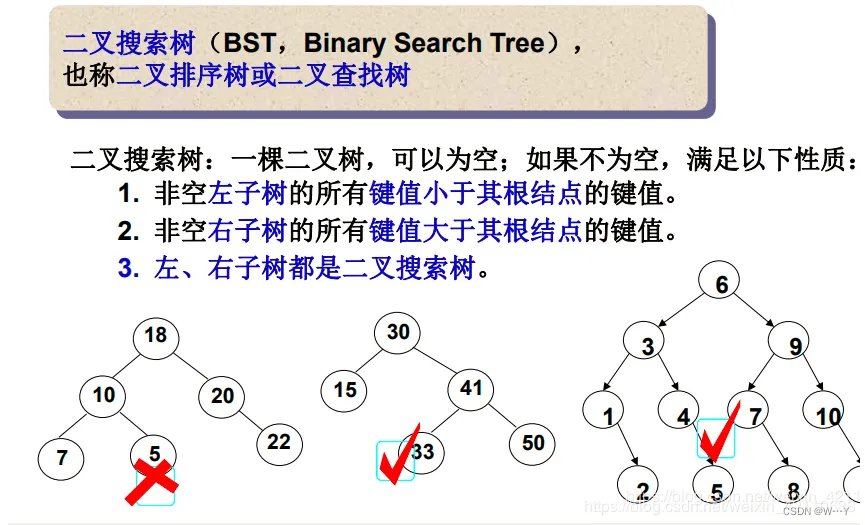
1.1 二叉搜索树概念
1.2 二叉搜索树操作
1.3 二叉搜索树的实现
1.4 二叉搜索树的应用
1.5 二叉搜索树的性能分析
1. 二叉搜索树
1.1 二叉搜索树概念
二叉搜索树又称二叉排序树,它或者是一棵空树,或者是具有以下性质的二叉树:
若它的左子树不为空,则左子树上所有节点的值都小于根节点的值
若它的右子树不为空,则右子树上所有节点的值都大于根节点的值
它的左右子树也分别为二叉搜索树
1.2 二叉搜索树操作
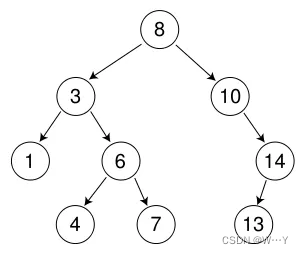
int a[] = {8, 3, 1, 10, 6, 4, 7, 14, 13};1. 二叉搜索树的查找
a、从根开始比较,查找,比根大则往右边走查找,比根小则往左边走查找。
b、最多查找高度次,走到到空,还没找到,这个值不存在。
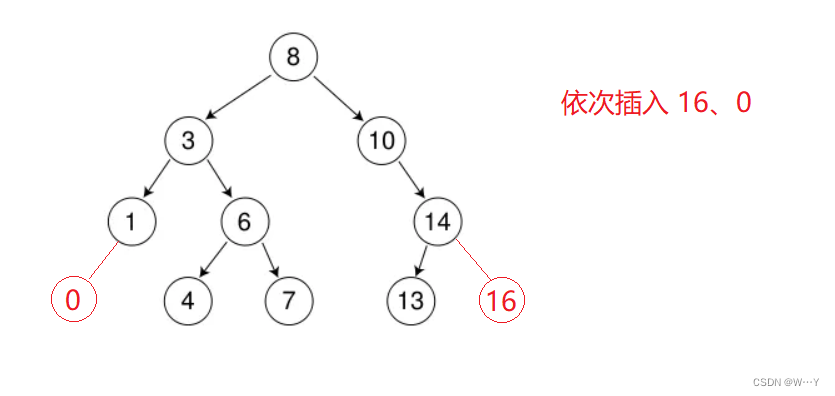
2. 二叉搜索树的插入
插入的具体过程如下:
a. 树为空,则直接新增节点,赋值给root指针
b. 树不空,按二叉搜索树性质查找插入位置,插入新节点
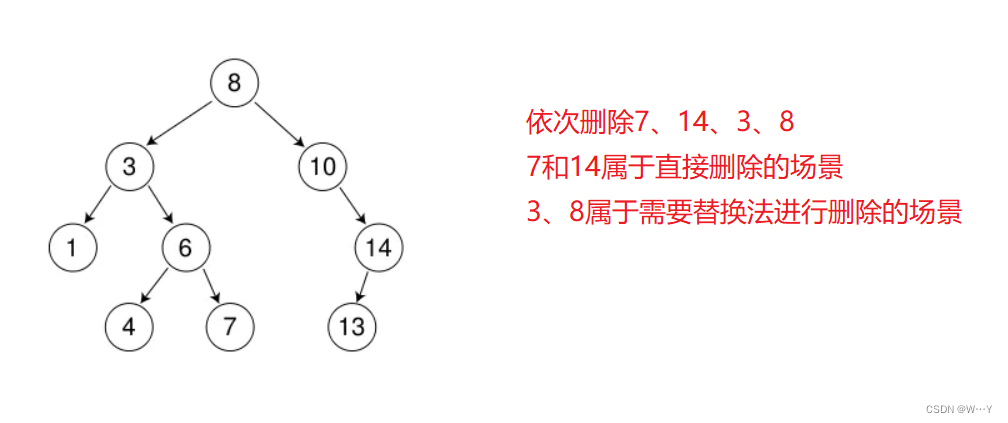
1. **二叉搜索树的删除
首先查找元素是否在二叉搜索树中,如果不存在,则返回, 否则要删除的结点可能分下面四种情
况:
a. 要删除的结点无孩子结点
b. 要删除的结点只有左孩子结点
c. 要删除的结点只有右孩子结点
d. 要删除的结点有左、右孩子结点
看起来有待删除节点有4中情况,实际情况a可以与情况b或者c合并起来,因此真正的删除过程
如下:
情况b:删除该结点且使被删除节点的双亲结点指向被删除节点的左孩子结点--直接删除
情况c:删除该结点且使被删除节点的双亲结点指向被删除结点的右孩子结点--直接删除
情况d:在它的右子树中寻找中序下的第一个结点(关键码最小),用它的值填补到被删除节点
中,再来处理该结点的删除问题--替换法删除
所以我们要进行二叉搜索树的增删查改。
1.3 二叉搜索树的实现
template<class k>
struct BSTreeNode
{
typedef BSTreeNode<k> Node;
Node* _left;
Node* _right;
k _key;
BSTreeNode(const k& key)
:_left(nullptr)
,_right(nullptr)
,_key(key)
{}
};创建一个树节点的类模板进行封装,const T& data = T()是对_data参数进行匿名构造缺省值,所以它会去调用其自己的默认构造函数。将左右两个指针置空即可。
template<class k>
class BSTree
{
public:
typedef BSTreeNode<k> Node;
//使用了强制生成默认构造!!!
BSTree() = default;
BSTree(const BSTree<k>& t)
{
_root = Copy(t._root);
}
BSTree<k>& operator=(BSTree<k> t)
{
swap(_root, t._root);
return *this;
}
~BSTree()
{
Destroy(_root);
}
bool Insert(const k& key)
{
if (_root == nullptr)
{
_root = new Node(key);
return true;
}
Node* parent = nullptr;
Node* cur = _root;
while (cur)
{
if (cur->_key > key)
{
parent = cur;
cur = cur->_left;
}
else if(cur->_key < key)
{
parent = cur;
cur = cur->_right;
}
else
{
return false;
}
}
cur = new Node(key);
if (parent->_key > key)
{
parent->_left = cur;
}
else
{
parent->_right = cur;
}
return true;
}
bool Find(const k& key)
{
Node* cur = _root;
while (cur)
{
if (cur->_key > key)
{
cur = cur->_left;
}
else if (cur->_key < key)
{
cur = cur->_right;
}
else
{
return true;
}
}
return false;
}
bool Erase(const k& key)
{
// 如果树为空,删除失败
if (nullptr == _root)
return false;
Node* parent = nullptr;
Node* cur = _root;
while (cur)
{
if (cur->_key > key)
{
parent = cur;
cur = cur->_left;
}
else if (cur->_key < key)
{
parent = cur;
cur = cur->_right;
}
else
{
if (cur->_left == nullptr)
{
if (cur == _root)
{
_root = cur->_right;
}
else
{
if (cur == parent->_left)
{
parent->_left = cur->_right;
}
else
{
parent->_right = cur->_right;
}
}
delete cur;
return true;
}
else if (cur->_right == nullptr)
{
if (cur == _root)
{
_root = cur->_left;
}
else
{
if (parent->_left == cur)
{
parent->_left = cur->_left;
}
else
{
parent->_right = cur->_left;
}
}
delete cur;
return true;
}
else
{
Node* rightminparent = cur;
Node* rightmin = cur->_right;
while (rightmin->_left)
{
rightminparent = rightmin;
rightmin = rightmin->_left;
}
cur->_key = rightmin->_key;
if (rightminparent->_left == rightmin)
{
rightminparent->_left = rightmin->_right;
}
else
{
rightminparent->_right = rightmin->_right;
}
delete rightmin;
return true;
}
}
}
return false;
}
void InOrder()
{
_InOrder(_root);
cout << endl;
}
bool FindR(const k& key)
{
return _FindR(_root, key);
}
bool InsertR(const k& key)
{
return _InsertR(_root, key);
}
bool EraseR(const k& key)
{
return _EraseR(_root, key);
}
private:
void Destroy(Node* root)
{
if (root == nullptr)
return;
Destroy(root->_left);
Destroy(root->_right);
delete root;
}
Node* Copy(Node* root)
{
if (root == nullptr)
return nullptr;
Node* newRoot = new Node(root->_key);
newRoot->_left = Copy(root->_left);
newRoot->_right = Copy(root->_right);
return newRoot;
}
void _InOrder(Node* root)
{
if (root == nullptr)
{
return;
}
_InOrder(root->_left);
cout << root->_key << " ";
_InOrder(root->_right);
}
bool _FindR(Node* root, const k& key)
{
if (root == nullptr)
{
return false;
}
if (root->_key > key)
{
_FindR(root->_left, key);
}
else if (root->_key < key)
{
_FindR(root->_right, key);
}
else
{
return true;
}
}
bool _EraseR(Node*& root, const k& key)
{
if (root == nullptr)
{
return false;
}
if (root->_key < key)
{
return _EraseR(root->_left, key);
}
else if (root->_key < key)
{
return _EraseR(root->_right, key);
}
else
{
Node* del = root;
if (root->_right == nullptr)
{
root = root->_left;
}
else if (root->_left == nullptr)
{
root = root->_right;
}
else
{
Node* rightmin = root->_right;
while (root->_left)
{
rightmin = rightmin->_left;
}
swap(root->_key, rightmin->_key);
return _EarseR(root->_right, key);
}
delete del;
return true;
}
}
bool _InsertR(Node*& root, const k& key)
{
if (root == nullptr)
{
root = new Node(key);
return true;
}
if (root->_key < key)
{
return _InsertR(root->_right, key);
}
else if (root->_key > key)
{
return _InsertR(root->_left, key);
}
else
{
return false;
}
}
Node* _root = nullptr;
};InsertR、FindR、EarseR 函数分别是使用递归的插入、查询、删除。没有R的是其正常的模拟实现。
因为在类的公有层面,我们在主函数时去调用其对应的函数时,其函数隐藏了一个默认参数this指针,所以我们为了去调用时方便,在私有又套了一层其函数。
1.4 二叉搜索树的应用
1. K模型:K模型即只有key作为关键码,结构中只需要存储Key即可,关键码即为需要搜索到
的值。
比如:给一个单词word,判断该单词是否拼写正确,具体方式如下:
以词库中所有单词集合中的每个单词作为key,构建一棵二叉搜索树
在二叉搜索树中检索该单词是否存在,存在则拼写正确,不存在则拼写错误。
2. KV模型:每一个关键码key,都有与之对应的值Value,即<Key, Value>的键值对。该种方
式在现实生活中非常常见:
比如英汉词典就是英文与中文的对应关系,通过英文可以快速找到与其对应的中文,英
文单词与其对应的中文<word, chinese>就构成一种键值对;
再比如统计单词次数,统计成功后,给定单词就可快速找到其出现的次数,单词与其出
现次数就是<word, count>就构成一种键值对。
改造二叉搜索树为KV结构 ,我们只需要将其前面的代码进行修改即可为KV模型。
namespace key_value
{
template<class K, class V>
struct BSTreeNode
{
typedef BSTreeNode<K, V> Node;
Node* _left;
Node* _right;
K _key;
V _value;
BSTreeNode(const K& key, const V& value)
:_left(nullptr)
,_right(nullptr)
,_key(key)
,_value(value)
{}
};
template<class K, class V>
class BSTree
{
typedef BSTreeNode<K, V> Node;
public:
bool Insert(const K& key, const V& value)
{
if (_root == nullptr)
{
_root = new Node(key, value);
return true;
}
Node* parent = nullptr;
Node* cur = _root;
while (cur)
{
if (cur->_key < key)
{
parent = cur;
cur = cur->_right;
}
else if (cur->_key > key)
{
parent = cur;
cur = cur->_left;
}
else
{
return false;
}
}
cur = new Node(key, value);
if (parent->_key < key)
{
parent->_right = cur;
}
else
{
parent->_left = cur;
}
return true;
}
Node* Find(const K& key)
{
Node* cur = _root;
while (cur)
{
if (cur->_key < key)
{
cur = cur->_right;
}
else if (cur->_key > key)
{
cur = cur->_left;
}
else
{
return cur;
}
}
return nullptr;
}
bool Erase(const K& key)
{
Node* parent = nullptr;
Node* cur = _root;
while (cur)
{
if (cur->_key < key)
{
parent = cur;
cur = cur->_right;
}
else if (cur->_key > key)
{
parent = cur;
cur = cur->_left;
}
else
{
if (cur->_left == nullptr)
{
if (cur == _root)
{
_root = cur->_right;
}
else
{
if (cur == parent->_right)
{
parent->_right = cur->_right;
}
else
{
parent->_left = cur->_right;
}
}
delete cur;
return true;
}
else if (cur->_right == nullptr)
{
if (cur == _root)
{
_root = cur->_left;
}
else
{
if (cur == parent->_right)
{
parent->_right = cur->_left;
}
else
{
parent->_left = cur->_left;
}
}
delete cur;
return true;
}
else
{
// 替换法
Node* rightMinParent = cur;
Node* rightMin = cur->_right;
while (rightMin->_left)
{
rightMinParent = rightMin;
rightMin = rightMin->_left;
}
cur->_key = rightMin->_key;
if (rightMin == rightMinParent->_left)
rightMinParent->_left = rightMin->_right;
else
rightMinParent->_right = rightMin->_right;
delete rightMin;
return true;
}
}
}
return false;
}
void InOrder()
{
_InOrder(_root);
cout << endl;
}
private:
void _InOrder(Node* root)
{
if (root == nullptr)
return;
_InOrder(root->_left);
cout << root->_key << " ";
_InOrder(root->_right);
}
private:
Node* _root = nullptr;
};
}1.5 二叉搜索树的性能分析
插入和删除操作都必须先查找,查找效率代表了二叉搜索树中各个操作的性能。
对有n个结点的二叉搜索树,若每个元素查找的概率相等,则二叉搜索树平均查找长度是结点在二
叉搜索树的深度的函数,即结点越深,则比较次数越多。
但对于同一个关键码集合,如果各关键码插入的次序不同,可能得到不同结构的二叉搜索树:
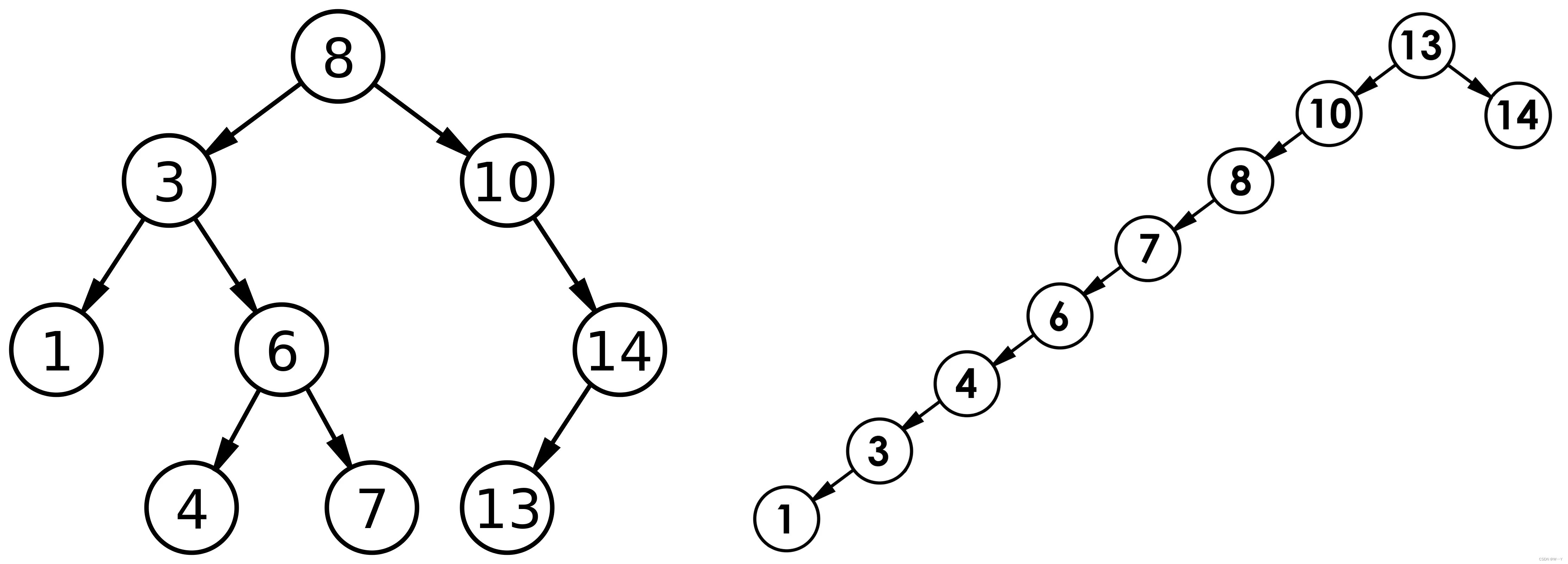 最优情况下,二叉搜索树为完全二叉树(或者接近完全二叉树),其平均比较次数为:log_2n
最优情况下,二叉搜索树为完全二叉树(或者接近完全二叉树),其平均比较次数为:log_2n
最差情况下,二叉搜索树退化为单支树(或者类似单支),其平均比较次数为:
如果退化成单支树,二叉搜索树的性能就失去了。那能否进行改进,不论按照什么次序插
入关键码,二叉搜索树的性能都能达到最优?那么我们后续学习的AVL树和红黑树就可以上
场了。
以上就是全部内容,感谢大家观看!!!
