当前位置：首页 > article >正文

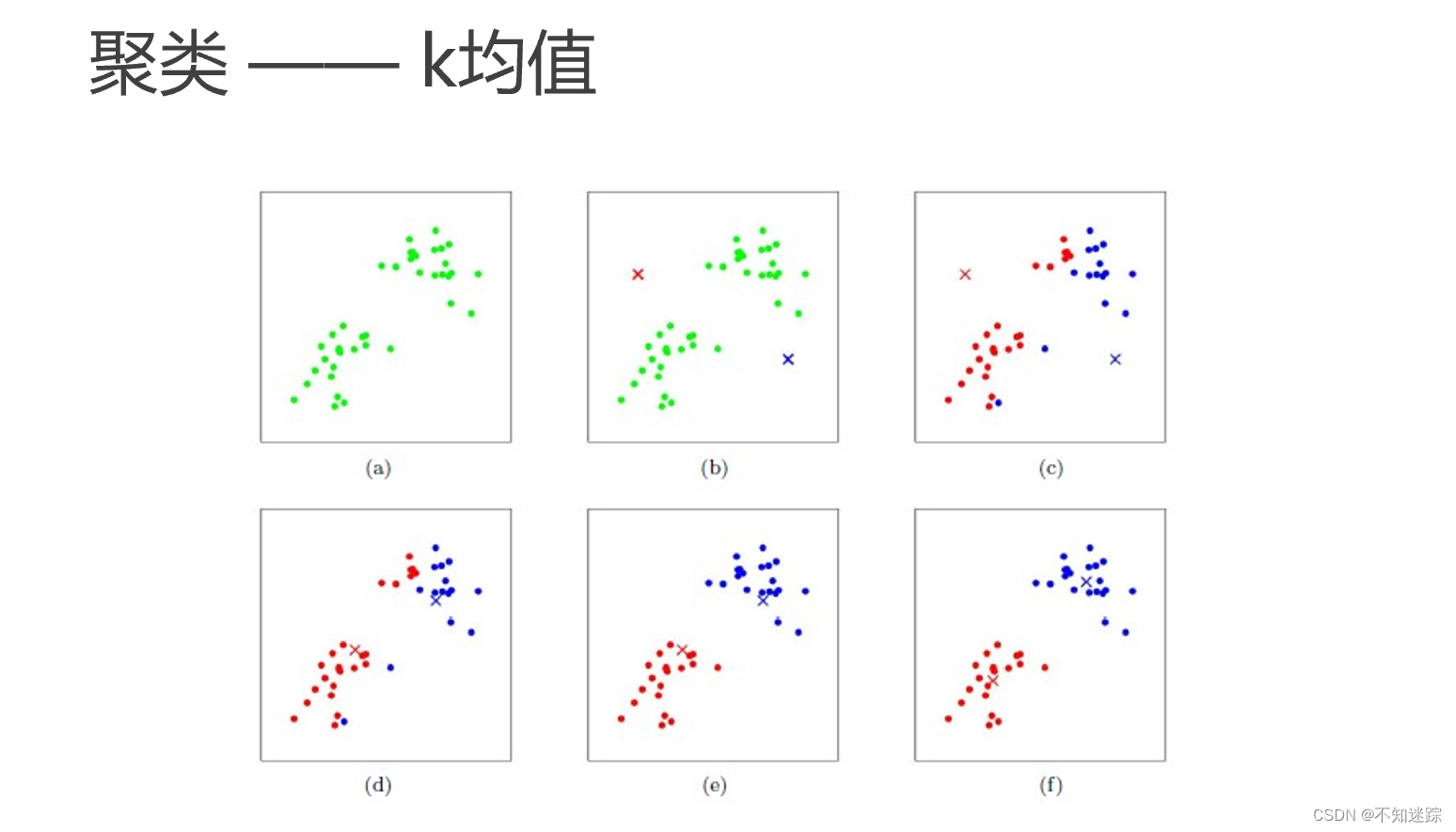
[机器学习]K-means——聚类算法

article 2024/11/19 19:31:42

一.K-means算法概念

二.代码实现

# 0. 引入依赖
import numpy as np
import matplotlib.pyplot as plt # 画图依赖
from sklearn.datasets import make_blobs # 从sklearn中直接生成聚类数据

# 1. 数据加载
# 生成（n_samples：样本点，centers：中心点，random_state：随机种子，cluster_std：聚类标准差）
x, y = make_blobs( n_samples=100, centers=6, random_state=1234, cluster_std=0.6 )
"""
print(x)
# 画原始图
plt.figure(figsize=(6,6))
plt.scatter(x[:,0], x[:,1], c=y)
plt.show()
"""

# 2. 算法实现
# 引入scipy中的距离函数，默认欧式距离
from scipy.spatial.distance import cdist

class K_Means(object):
    # 初始化，每一类均值参数：n_clusters（K），迭代次数：max_iter，初始质心：centroids
    def __init__(self, n_clusters=6, max_iter=300, centroids=[]):
        self.n_clusters = n_clusters
        self.max_iter = max_iter
        self.centroids = np.array(centroids, dtype=np.float64)

    # 训练模型方法，k-means聚类过程，传入原始数据(x本身就有两个坐标)
    def fit(self, data):
        # 假如没有指定初始质心，就随机选取data中的点作为初始质心
        if (len(self.centroids) == 0):
            # 从data中随机生成0到data行数的6个整数，作为索引值
            # 从0到len(data)中随机选取n_clusters个
            self.centroids = data[np.random.randint(0, len(data), self.n_clusters), :]

        # 开始迭代
        for i in range(self.max_iter):
            # 1. 计算距离矩阵，得到的是一个100*6的矩阵，每一行为该样本点到6个质心的距离
            distances = cdist(data, self.centroids)

            # 2. 对距离按有近到远排序，选取最近的质心点的类别，作为当前点的分类
            c_ind = np.argmin(distances, axis=1)

            # 3. 对每一类数据进行均值计算，更新质心点坐标
            for i in range(self.n_clusters):
                # 排除掉没有出现在c_ind里的类别
                if i in c_ind:
                    # 选出所有类别是i的点，取data里面坐标的均值，更新第i个质心
                    self.centroids[i] = np.mean(data[c_ind == i], axis=0)

    # 实现预测方法
    def predict(self, samples):
        # 跟上面一样，先计算距离矩阵，然后选取距离最近的那个质心的类别
        distances = cdist(samples, self.centroids)
        c_ind = np.argmin(distances, axis=1)

        return c_ind

"""
dist = np.array([[121, 221, 32, 43],
                 [121, 1, 12, 23],
                 [65, 21, 2, 43],
                 [1, 221, 32, 43],
                 [21, 11, 22, 3], ])
c_ind = np.argmin(dist, axis=1)
print(c_ind)
x_new = x[0:5]
print(x_new)
print(c_ind == 2)
print(x_new[c_ind == 2])
np.mean(x_new[c_ind == 2], axis=0)
"""

# 3. 测试
# 定义一个绘制子图函数
def plotKMeans(x, y, centroids, subplot, title):
    # 分配子图，121表示1行2列的子图中的第一个
    plt.subplot(subplot)
    plt.scatter(x[:,0], x[:,1], c='red')
    # 画出质心点
    plt.scatter(centroids[:,0], centroids[:,1], c=np.array(range(6)), s=100)
    plt.title(title)

# centroids指定质心初始点
kmeans = K_Means(max_iter=300, centroids=np.array([[2,1],[2,2],[2,3],[2,4],[2,5],[2,6]]))

plt.figure(figsize=(16, 6))
plotKMeans( x, y, kmeans.centroids, 121, 'Initial State' )

# 开始聚类
kmeans.fit(x)

plotKMeans( x, y, kmeans.centroids, 122, 'Final State' )

# 预测新数据点的类别
x_new = np.array([[0,0],[10,7]])
y_pred = kmeans.predict(x_new)

print("经过训练的质心为：",kmeans.centroids)
print("这些点的预测为：",y_pred)

plt.scatter(x_new[:,0], x_new[:,1], s=100, c='black')

plt.show()

经过训练的质心为： [[ 5.76444812 -4.67941789]
[-2.89174024 -0.22808556]
[-5.89115978 2.33887408]
[-4.53406813 6.11523454]
[-1.15698106 5.63230377]
[ 9.20551979 7.56124841]]
这些点的预测为： [1 5]