三、搜索与图论

DFS

排列数字

#include<iostream>
using namespace std;
const int N = 10;
int a[N], b[N];
int n;

void dfs(int u){
    if(u > n){
        for(int i = 1; i <= n; i++)
            cout<<a[i]<<" ";
        cout<<endl;
        return;
    }
    for(int i = 1; i <= n; i++){
        if(!b[i]){
            b[i] = 1;
            a[u] = i;
            dfs(u + 1);
            b[i] = 0;
        }
    }
}

int main(){
    cin>>n;
    dfs(1);
    return 0;
}

n-皇后问题

#include<iostream>
using namespace std;
const int N = 20;
char g[N][N];
int a[N], b[N], c[N];
int n;

void dfs(int u){
    if(u > n){
        for(int i = 1; i <= n; i++){
            for(int j = 1; j <= n; j++)
                cout<<g[i][j];
            cout<<endl;
        }
        cout<<endl;
        return;
    }
    for(int i = 1; i <= n; i++){
        if(!a[i] && !b[u + i] && !c[-u + i + n]){
            a[i] = b[u + i] = c[-u + i + n] = 1;
            g[u][i] = 'Q';
            dfs(u + 1);
            g[u][i] = '.';
            a[i] = b[u + i] = c[-u + i + n] = 0;
        }
    }
}

int main(){
    cin>>n;
    for(int i = 1; i <= n; i++)
        for(int j = 1; j <= n; j++)
            g[i][j] = '.';
    dfs(1);
    return 0;
}

BFS

走迷宫

#include<iostream>
#include<cstring>
using namespace std;
const int N = 110;
int g[N][N], d[N][N];
pair<int, int> q[N * N];
int hh, tt = - 1;
int n, m;

int dx[] = {0, 0, 1, -1};
int dy[] = {1, -1, 0, 0};

void bfs(int x, int y){
    memset(d, -1, sizeof(d));
    q[++tt] = make_pair(x, y);
    d[x][y] = 0;
    while(hh <= tt){
        auto t = q[hh++];
        for(int i = 0; i < 4; i++){
            int a = dx[i] + t.first, b = dy[i] + t.second;
            if(a < 1 || a > n || b < 1 || b > m) continue;
            if(d[a][b] != -1) continue;
            if(g[a][b] != 0) continue;
            d[a][b] = d[t.first][t.second] + 1;
            q[++tt] = make_pair(a, b);
        }
    }
    cout<<d[n][m];
}

int main(){
    cin>>n>>m;
    for(int i = 1; i <= n; i++)
        for(int j = 1; j <= m; j++)
            cin>>g[i][j];
    bfs(1, 1);
    return 0;
} 

八数码

#include<iostream>
#include<unordered_map>
using namespace std;
const int N = 1e6; //一共有9!种情况
unordered_map<string, int> d;
string q[N];
int hh, tt = -1;
int n = 9;

int dx[] = {0, 0, 1, -1};
int dy[] = {1, -1, 0, 0};

int bfs(string s){
    q[++tt] = s;
    d[s] = 0;
    //记录终点
    string end = "12345678x";
    while(hh <= tt){
        string t = q[hh++];
        //存储当前位置到起点的距离
        int dis = d[t];
        //如果到终点了，那就返回距起点距离
        if(t == end) return dis;
        //查找x的下标
        int k = t.find('x');
        //x在矩阵中的位置
        int x = k / 3, y = k % 3;
        for(int i = 0; i < 4; i++){
            int a = x + dx[i], b = y + dy[i];
            if(a < 0 || a > 2 || b < 0 || b > 2) continue;
            //转移x
            swap(t[k], t[3 * a + b]);
            //如果没有遍历过，那就存储到队列中
            if(!d.count(t)){
                d[t] = dis + 1;
                q[++tt] = t;
            }
            //还原
            swap(t[k], t[3 * a + b]);
        }
    }
    return -1;
}

int main(){
    char c;
    string s = "";
    for(int i = 0; i < n; i++){
        cin>>c;
        s += c;
    }
    cout<<bfs(s);
    return 0;
}

树和图的存储

树是一种特殊的图
存储可以用链式向前星或者vector

//链式向前星
#include<iostream>
#include<cstring>
using namespace std;
const int N = 1e5 + 10, M = 2 * N;
int h[N], e[N], ne[N], idx;
int st[N];

void add(int a, int b){
    e[idx] = b;
    ne[idx] = h[a];
    h[a] = idx;
    idx++;
}

void dfs(int u){
    st[u] = 1;
    for(int i = u; i != -1; i = ne[i]){
        int j = e[i];
        if(!st[j]) dfs(j);
    }
}

int main(){
    memset(h, -1, sizeof(h));
    return 0;
}

//vector存储
#include<iostream>
#include<vector>
using namespace std;
const int N = 1e5 + 10;
vector<int> v[N];
int st[N];

void add(int a, int b){
    v[a].push_back(b);
    v[b].push_back(a);
}

void dfs(int u){
    st[u] = 1;
    for(int i = 0; i < v[u].size(); i++){
        int j = v[u][i];
        if(!st[j]) dfs(j);
    }
}

int main(){
    return 0;
}

树与图的深度优先遍历

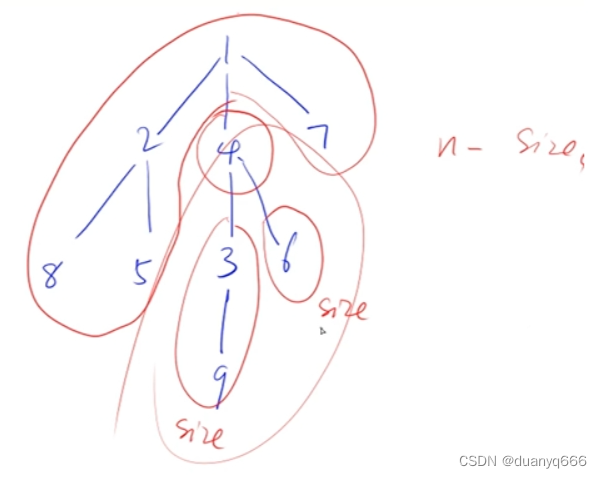
树的重心

#include<iostream>
#include<cstring>
using namespace std;
const int N = 1e5 + 10, M = 2 * N;
int h[N], e[M], ne[M], idx;
int st[N];
int n, ans = 1e9;

void add(int a, int b){
    e[idx] = b;
    ne[idx] = h[a];
    h[a] = idx;
    idx++;
}

int dfs(int u){
    st[u] = 1;
    //cnt存储以u为根的节点数（包括u），res是删除掉某个节点后的最大连通子图节点数
    int cnt = 1, res = 0; 
    for(int i = h[u]; i != -1; i = ne[i]){
        int j = e[i];
        if(!st[j]){
            //以u为节点的单棵子树的节点数
            int t = dfs(j);
            //计算以j为根的树的节点数
            cnt += t;
            //记录最大连通子图节点数
            res = max(res, t);
        }
    }
    //以u为重心，最大的连通子图节点数
    res = max(res, n - cnt);
    ans = min(ans, res);
    return cnt;
}

int main(){
    memset(h, -1, sizeof(h));
    cin>>n;
    int a, b;
    for(int i = 0; i < n - 1; i++){
        cin>>a>>b;
        add(a, b);
        add(b, a);
    }
    dfs(1);
    cout<<ans;
    return 0;
}

树与图的宽度优先遍历

图中点的层次

#include<iostream>
#include<cstring>
using namespace std;
const int N = 1e5 + 10, M = 2 * N;
int h[N], e[M], ne[M], idx;
int q[N], d[N], hh, tt = -1;
int n, m;

void add(int a, int b){
    e[idx] = b;
    ne[idx] = h[a];
    h[a] = idx;
    idx++;
}

void bfs(int u){
    memset(d, -1, sizeof(d));
    q[++tt] = u;
    d[u] = 0;
    while(hh <= tt){
        //使用队头，弹出队头
        int t = q[hh++];
        for(int i = h[t]; i != -1; i = ne[i]){
            int j = e[i];
            if(d[j] == -1){
                //更新距离
                d[j] = d[t] + 1;
                //入队
                q[++tt] = j;
            }
        }
    }
    cout<<d[n];
}

int main(){
    memset(h, -1, sizeof(h));
    cin>>n>>m;
    int x, y;
    while(m--){
        cin>>x>>y;
        add(x, y);
    }
    bfs(1);
    return 0;
}

拓扑排序

有向无环图也是拓扑图
入度：有多少条边指向自己
出度：有多少条边出去

有向图的拓扑序列

入度为0就是起点，出度为0就是终点

#include<iostream>
#include<cstring>
using namespace std;
const int N = 1e5 + 10;
int h[N], e[N], ne[N], idx;
int q[N], hh, tt = -1;
int n, m;
int r[N]; //存储入度

void add(int a, int b){
    e[idx] = b;
    ne[idx] = h[a];
    h[a] = idx;
    idx++;
}

void bfs(){
    //判断哪些点入度为0
    for(int i = 1; i <= n; i++)
        if(!r[i]) q[++tt] = i;
    while(hh <= tt){
        int t = q[hh++];
        for(int i = h[t]; i != -1; i = ne[i]){
            int j = e[i];
            r[j]--;
            if(!r[j]) q[++tt] = j;
        }
    }
    if(tt == n - 1){
        for(int i = 0; i <= tt; i++) cout<<q[i]<<" ";
    }else cout<<-1;
}

int main(){
    memset(h, -1, sizeof(h));
    cin>>n>>m;
    int x, y;
    while(m--){
        cin>>x>>y;
        add(x, y);
        r[y]++;
    }
    bfs();
    return 0;
}

最短路

帮助理解

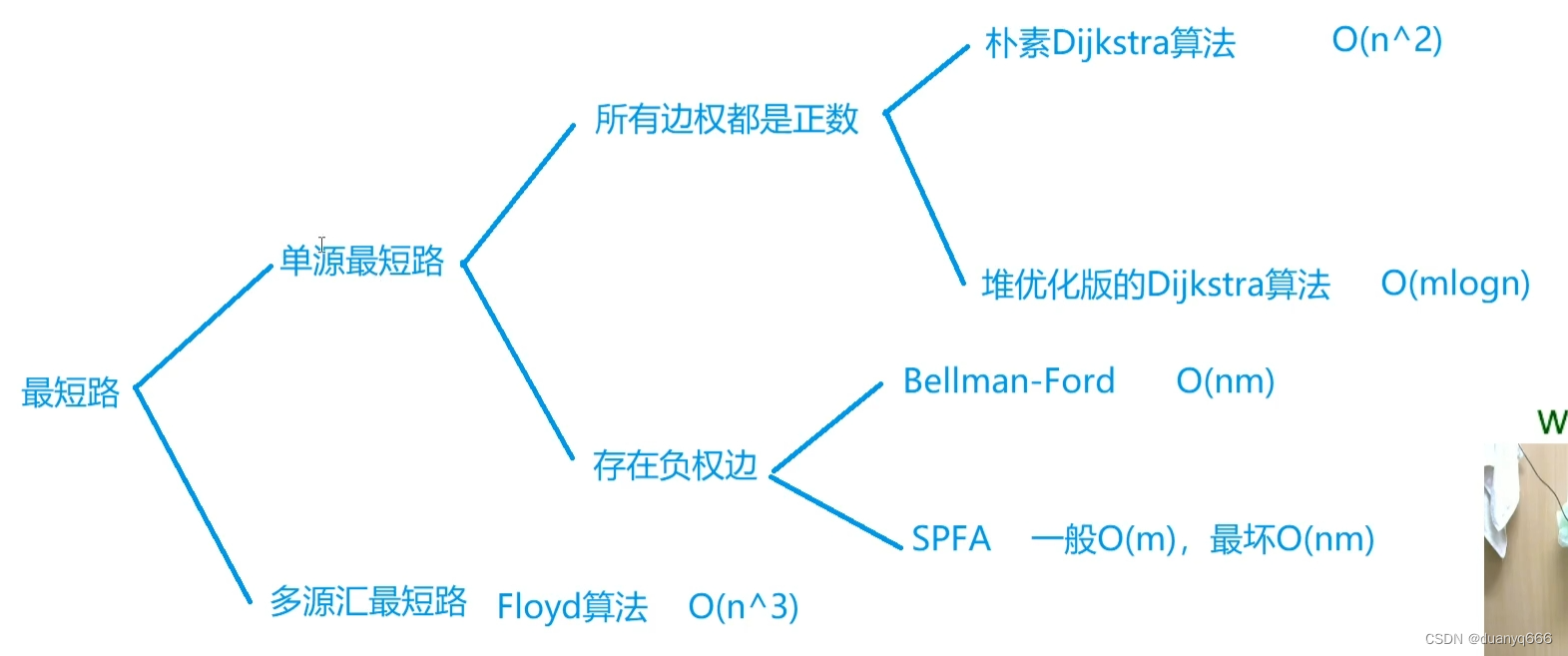
Dijkstra

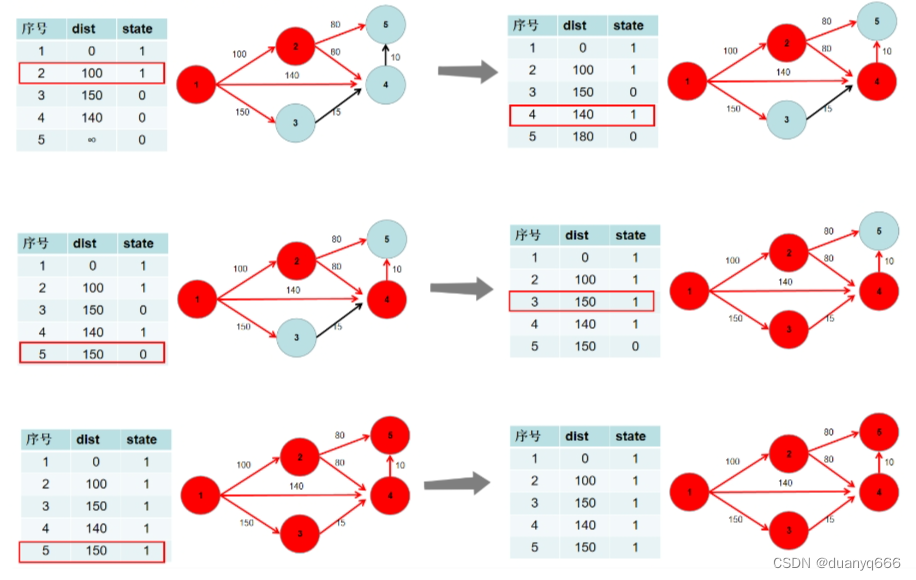
Dijkstra求最短路 I

#include<iostream>
#include<cstring>
using namespace std;
const int N = 510;
int g[N][N], d[N], b[N];
int n, m;

void dijkstra(int u){
    memset(d, 0x3f, sizeof(d));
    d[u] = 0;
    for(int i = 0; i < n; i++){
        int t = -1;
        for(int j = 1; j <= n; j++)
            if(!b[j] && (t == -1 || d[t] > d[j])) t = j;
        b[t] = 1;
        for(int j = 1; j <= n; j++)
            d[j] = min(d[j], d[t] + g[t][j]);
    }
    cout<<((d[n] == 0x3f3f3f3f) ? -1 : d[n]);
}

int main(){
    memset(g, 0x3f, sizeof(g));
    cin>>n>>m;
    int x, y, z;
    while(m--){
        cin>>x>>y>>z;
        g[x][y] = min(g[x][y], z);
    }
    dijkstra(1);
    return 0;
}

Dijkstra求最短路 II

#include<iostream>
#include<cstring>
#include<queue>
using namespace std;
const int N = 2e5;
int h[N], e[N], ne[N], w[N], idx; //w[i]存储上个点到i的距离
int d[N], b[N];
int n, m;
priority_queue<pair<int, int>, vector<pair<int, int>>, greater<pair<int, int>>> q; //小根堆，第一个元素存储距离，第二个元素存储下标

void add(int x, int y, int z){
    e[idx] = y;
    w[idx] = z;
    ne[idx] = h[x];
    h[x] = idx;
    idx++;
}

void dijkstra(int u){
    memset(d, 0x3f, sizeof(d));
    d[u] = 0;
    q.push(make_pair(0, 1));
    while(q.size()){
        auto t = q.top();
        q.pop();
        int x = t.first, y = t.second;
        if(b[y]) continue; //如果遍历过就退出
        b[y] = 1;
        for(int i = h[y]; i != -1; i = ne[i]){
            int j = e[i];
            if(d[j] > x + w[i]){
                d[j] = x + w[i];
                q.push(make_pair(d[j], j));
            }
        }
    }
    cout<<(d[n] == 0x3f3f3f3f ? -1 : d[n]);
}

int main(){
    memset(h, -1, sizeof(h));
    cin>>n>>m;
    int x, y, z;
    while(m--){
        cin>>x>>y>>z;
        add(x, y, z);
    }
    dijkstra(1);
    return 0;
}

增加点权，求有多少条最短路

题目链接

#include<iostream>
#include<cstring>
using namespace std;
int g[505][505], dis[505], st[505];
int a[505], paths[505], teams[505];
int n, m, c1, c2;

void dj(int u){
    teams[u] = a[u];
    paths[u] = 1;
    dis[u] = 0;
    for(int j = 0; j < n; j++){
        int t = -1;
        for(int i = 0; i < n; i++){
            if(!st[i] && (t == -1 || dis[t] > dis[i])){
                t = i;
            }
        }
        st[t] = 1;
        for(int i = 0; i < n; i++){
            if(dis[i] > dis[t] + g[t][i]){
                dis[i] = dis[t] + g[t][i]; 
                paths[i] = paths[t]; //继承路径条数
                teams[i] = teams[t] + a[i]; //更新救援队人数
            }else if(dis[i] == dis[t] + g[t][i]){
                if(teams[i] < teams[t] + a[i]){
                    teams[i] = teams[t] + a[i]; //选救援队人数更多的
                } 
                paths[i] += paths[t]; //累加路径条数
            }
        }
    }
}

int main(){
    memset(g, 0x3f, sizeof(g));
    cin>>n>>m>>c1>>c2;
    for(int i = 0; i < n; i++) cin>>a[i];
    while(m--){
        int x, y, z;
        cin>>x>>y>>z;
        g[x][y] = g[y][x] = min(g[x][y], z);
    }
    memset(dis, 0x3f, sizeof(dis));
    dj(c1);
    cout<<paths[c2]<<" "<<teams[c2];
    return 0;
}

增加边权，求花费最少

题目链接

#include<iostream>
#include<cstring>
#include<vector>
using namespace std;
int g[505][505], dis[505], st[505];
int cost[505][505], c[505], pre[505];
vector<int> path;
int n, m, s, d;

void dj(int u){
    dis[u] = 0;
    c[u] = 0;
    for(int i = 0; i < n; i++){
        int t = -1;
        for(int j = 0; j < n; j++){
            if(!st[j] && (t == -1 || dis[t] > dis[j])){
                t = j;
            }
        }
        st[t] = 1;
        for(int j = 0; j < n; j++){
            if(dis[j] > dis[t] + g[t][j]){
            	pre[j] = t;
                dis[j] = dis[t] + g[t][j];
                c[j] = c[t] + cost[t][j];
            }else if(dis[j] == dis[t] + g[t][j] && c[j] > c[t] + cost[t][j]){
            	pre[j] = t;
                c[j] = c[t] + cost[t][j];
            }
        }
    }
}

int main(){
    memset(g, 0x3f, sizeof(g));
    memset(dis, 0x3f, sizeof(dis));
    memset(c, 0x3f, sizeof(c));
    memset(cost, 0x3f, sizeof(cost));
    cin>>n>>m>>s>>d;
    while(m--){
        int x, y, z, h;
        cin>>x>>y>>z>>h;
        g[x][y] = g[y][x] = min(g[x][y], z);
        cost[x][y] = cost[y][x] = min(cost[x][y], h);
    }
    for(int i = 0; i < n; i++) pre[i] = i;
    dj(s);
    int q = d;
    while(q != s){
    	path.push_back(q);
    	q = pre[q];
	}
	path.push_back(s);
	int p = path.size();
	for(int i = p - 1; i >= 0; i--) cout<<path[i]<<" ";
	cout<<dis[d]<<" "<<c[d];
    return 0;
}

bellman-ford

有边数限制的最短路

如果负环在1到n的路径上，那就不存在最短路

#include<iostream>
#include<cstring>
using namespace std;
const int N = 510, M = 1e4 + 10;
int d[N], b[N]; //b数组备份
int n, m, k;
struct E{
    int x, y, z;
}e[M];

void bellman_ford(int u){
    memset(d, 0x3f, sizeof(d));
    d[u] = 0;
    //最多k条边
    for(int i = 0; i < k; i++){
        //每次只更新一条串联路径，防止更新了多条串联路径
        memcpy(b, d, sizeof(d));
        for(int j = 0; j < m; j++){
            int x = e[j].x, y = e[j].y, z = e[j].z;
            d[y] = min(d[y], b[x] + z);
        }
    }
    if(d[n] > 0x3f3f3f3f / 2) cout<<"impossible";
    else cout<<d[n];
}

int main(){
    cin>>n>>m>>k;
    int x, y, z;
    for(int i = 0; i < m; i++){
        cin>>x>>y>>z;
        e[i] = {x, y, z};
    }
    bellman_ford(1);
    return 0;
}

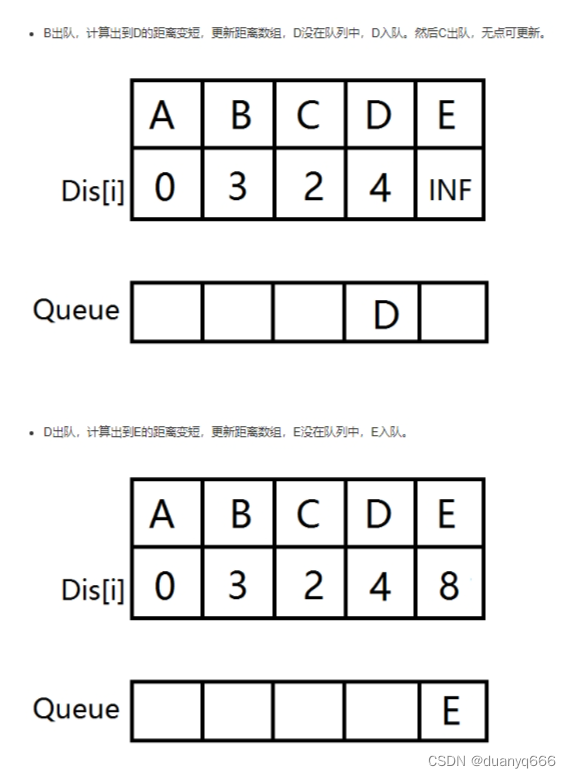
spfa

spfa求最短路

#include<iostream>
#include<cstring>
#include<queue>
using namespace std;
const int N = 1e5 + 10;
int h[N], e[N], ne[N], w[N], idx;
int dis[N], st[N];
int q[N], hh, tt = -1;
int n, m;

void add(int x, int y, int z){
    e[idx] = y;
    w[idx] = z;
    ne[idx] = h[x];
    h[x] = idx;
    idx++;
}

void spfa(int u){
    memset(dis, 0x3f, sizeof(dis));
    dis[u] = 0;
    q[++tt] = u;
    st[u] = 1;
    while(hh <= tt){
        int t = q[hh++];
        //有环，所以可能一个点会遍历两次
        st[t] = 0;
        for(int i = h[t]; i != -1; i = ne[i]){
            int j = e[i];
            if(dis[j] > dis[t] + w[i]){
                dis[j] = dis[t] + w[i];
                if(!st[j]){
                    q[++tt] = j;
                    st[j] = 1;
                }
            }
        }
    }
    if(dis[n] == 0x3f3f3f3f) cout<<"impossible";
    else cout<<dis[n];
}

int main(){
    memset(h, -1, sizeof(h));
    cin>>n>>m;
    int x, y, z;
    for(int i = 0; i < m; i++){
        cin>>x>>y>>z;
        add(x, y, z);
    }
    spfa(1);
    return 0;
}

spfa判断负环

#include<iostream>
#include<cstring>
using namespace std;
const int N = 2e3 + 10, M = 1e4 + 10;;
int h[N], e[M], ne[M], w[M], idx;
int dis[N], st[N], cnt[N];
int q[N * N], hh, tt = -1; //有环的时候，一个元素可能会一直插入队列，所以要开N * N
int n, m;

void add(int x, int y, int z){
    e[idx] = y;
    w[idx] = z;
    ne[idx] = h[x];
    h[x] = idx;
    idx++;
}

void spfa(){
    //存在的负权回路，不一定从1开始
    for(int i = 1; i <= n; i++){
        q[++tt] = i;
        st[i] = 1;
    }
    while(hh <= tt){
        int t = q[hh++];
        //有环，所以可能一个点会遍历两次
        st[t] = 0;
        for(int i = h[t]; i != -1; i = ne[i]){
            int j = e[i];
            if(dis[j] > dis[t] + w[i]){
                dis[j] = dis[t] + w[i];
                cnt[j] = cnt[t] + 1;
                if(cnt[j] >= n){
                    cout<<"Yes";
                    return;
                }
                if(!st[j]){
                    q[++tt] = j;
                    st[j] = 1;
                }
            }
        }
    }
    cout<<"No";
}

int main(){
    memset(h, -1, sizeof(h));
    cin>>n>>m;
    int x, y, z;
    for(int i = 0; i < m; i++){
        cin>>x>>y>>z;
        add(x, y, z);
    }
    spfa();
    return 0;
}

Floyd

Floyd求最短路

f(k, i, j) = f(k - 1, i, k) + f(k - 1, k, j);

#include<iostream>
using namespace std;
const int N = 210;
int f[N][N];
int n, m, k;

void floyd(){
    for(int k = 1; k <= n; k++)
        for(int i = 1; i <= n; i++)
            for(int j = 1; j <= n; j++)
                f[i][j] = min(f[i][j], f[i][k] + f[k][j]);
}

int main(){
    cin>>n>>m>>k;
    for(int i = 1; i <= n; i++)
        for(int j = 1; j <= n; j++)
            if(i == j) f[i][j] = 0;
            else f[i][j] = 0x3f3f3f3f;
    int x, y, z;
    for(int i = 1; i <= m; i++){
        cin>>x>>y>>z;
        f[x][y] = min(f[x][y], z);
    }
    floyd();
    for(int i = 1; i <= k; i++){
        cin>>x>>y;
        //可能存在负权边
        if(f[x][y] > 0x3f3f3f3f / 2) cout<<"impossible"<<endl;
        else cout<<f[x][y]<<endl;
    }
    return 0;
}

最小生成树

Prim

Kruskal

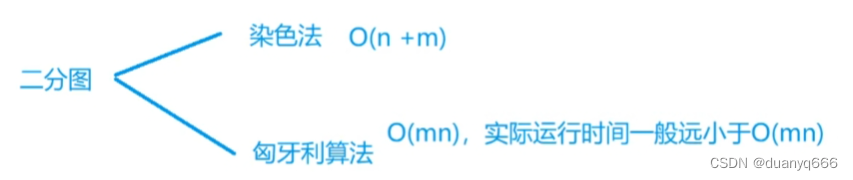
二分图

染色法判定二分图

匈牙利算法